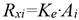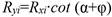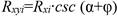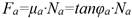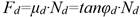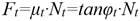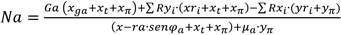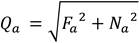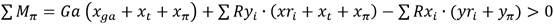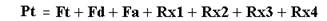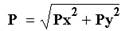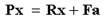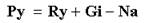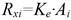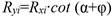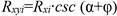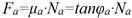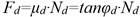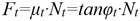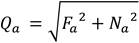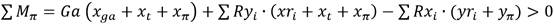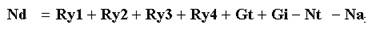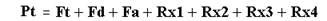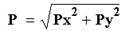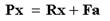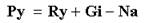Reyes-Suárez, Martínez-Rodríguez, Figal-Costales, Ramos-Carbajal, and Zaldívar-Fernández: Analysis of Suction and Stability of Implement in Localized Soil Preparation for Sugarcane Planting

INTRODUCTION

Sugarcane (Saccharum officinarum), is grown in tropical and subtropical regions, significantly increasing exports between 2000 and 2011, mainly in Brazil, which went from exporting 6.5 million tons in 2000 to 25.5 million tons in 2011 , being the largest world exporter of sugar, followed by Mexico, Guatemala and Colombia. In 2000, Cuba was the second largest exporter of sugar in the region, registering 3 500 000 t, falling in 2011 to 648 000 t according to FAO (2014). In recent years, Cuba showed a significant decreasing in sugar production, between other factors, due to low agricultural yields, for which it has been considered necessary to make changes in current agricultural practices (Herrera et al., 2003; Leyva et al., 2007; Betancourt et al., 2008). Among them, the change from the traditional sowing framework to the double row (Romer et al., 2009; Gómez et al., 2011; Ullah et al., 2016; Guru et al., 2017). At the same time, equipment are developed which are adapted to these new planting systems, including those of soil preparation for planting.

Cruz et al. (2018) state that, currently, the technologies used for soil preparation are conventional technology and conservationist. The latter is mainly based on localized preparation of soil, carried out exclusively in the zone of seedling root growth. It is a viable alternative, from the point of view of both, soil conservation and economy of energy resources devoted to the preparation of large areas of land.

The national project Increase in the effectiveness of mechanized processes in the production of sugarcane in the Base Business Unit "Héctor Molina Riaño", belongs to the program Development of the Sugar Agroindustry, run by the Agricultural Mechanization Center (CEMA). As part of this project, it is performed the task Development and evaluation of an implement of localized soil preparation. During the design process of this equipment, it is necessary to carry out certain analyses related to suction and stability, for which an analytical method and software have been developed to avoid the use of cumbersome traditional graphical methods, based on the elaboration of polygons of force and rays that make it difficult the evaluation of multiple variants. In the present work, the developed method and software is presented, through its application in a case study of the aggregate formed by a rubber wheel tractor YTO-1604 and a tillage implement with four staggered shanks. It is aimed at localized soil preparation for sugarcane sowing in double row furrows.

METHODS

Theoretical-Methodological Foundation

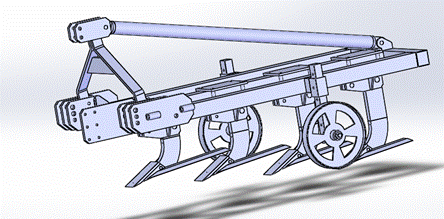
To develop the analytical method of calculation and the corresponding software, the mechanical-mathematical modeling of interaction of the aggregate with the soil was considered firstly, applying the laws of mechanics to the tractor-implement-soil system. Figure 1 shows an isometric view of the tillage implement object of study, where the staggering of four shanks with “V”- shaped blades that work at different depth can be seen, following the principle of the mechanical brush (Albóniga, 2011, 2015; Domínguez et al., 2014).

FIGURE 1

Isometric representation of the tillage implement having four staggered shanks object of study.

The stability conditions are evaluated by determining the loads that act on the wheels of the tractor, both rear and front. In this case, an analysis is made corresponding to a tractor with traction on the rear wheels. It is necessary to clarify that the analysis carried out corresponds to the longitudinal-vertical plane (x-y).

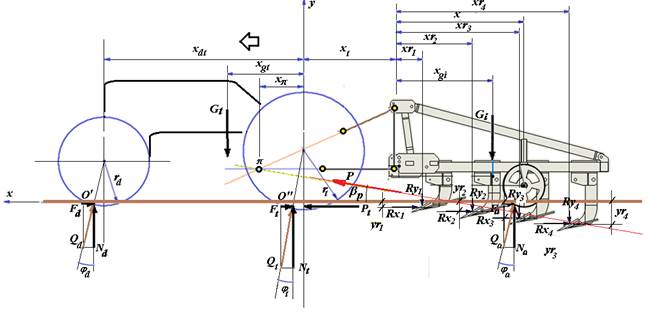
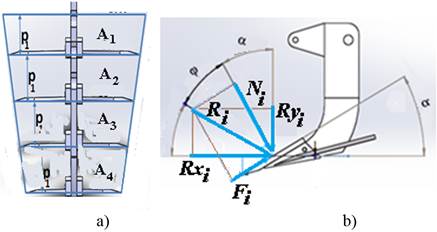
Figure 2 shows the system of forces acting on the aggregate formed by an YTO-1604 tractor with rubber wheels with traction on the rear wheels and the tillage implement having four staggered shanks with “V”- shaped blades.

FIGURE 2

System of forces that act on the aggregate tractor-tillage implement of staggered shanks.

For the achievement of a good "grip" or adhesion of the rear wheels, which enables the transmission of the power demanded in its interaction with the ground, a certain normal reaction force (Nt) in the rear wheel or motor is required (Figure 2) .(If this force does not reach a sufficient value, then wheel slippage may occur and the horizontal component (Pt) of the pulling force required for the work of the implement added to the tractor may not be transmitted. In this case, the work of the aggregate is considered unstable.

Of course, other parameters influence the ability of the driving wheel to transmit the power required through the soil. Among them, there is soil adherence and its state of compaction and humidity, as well as the design of the tires.

On the other hand, in relation to the front wheels, in order to guarantee a good steering of the tractor, an adequate load (Nd) is required on those wheels. It should not be excessive, but neither insufficient, since, in this case, it would be difficult to control the direction of the tractor and in the extreme case, lifting the front wheels. A stable work of the aggregate is complemented with an adequate "suction" of the implement that guarantees the natural penetration of the working implement on the ground with the suspension system working in a floating position. The model presented is implemented in such a way as to facilitate the evaluation of the influence of different parameters on the suction characteristics and stability of the system. Among the parameters that are taken as input variables, are the position of the support wheel, the angle of the wedges that make up the tillage implement of staggered shanks and the position of the instantaneous center of rotation of the tractor suspension system (point π in Figure 2). They, at the same time, constitute elements of design or regulation of the equipment. The forces and coordinates that appear in Figure 2 are the following:

- Force of draft required for the movement of the aggregate;

β _p

- angle that forms with the horizontal the resultant P of the tensile strength of the tillage implement;

- Horizontal component of the draft force;

Rx _i and Ry _i

- components of the resistance force that acts on each tillage unit;

Rxy

- result of the resistance forces of the soil to the action of the tillage unit;

Qa, Qt and Qd

-reactions of the ground on the support wheel of the implement, the rear wheel and the front wheel of the tractor, respectively;

Fa, Ft and Fd

-horizontal components of the reactions (friction by rolling) Qi;

Na, Nt and Nd

-normal components of the Qi reactions;

φa, φt and φd

-rolling resistance angles of the implement support wheel, the rear wheel of the tractor and the front wheel of the tractor, respectively;

Ga and Gt

- weights of the implement and of the tractor, respectively;

ra, rt and rd

-radiuses of the implement support wheel, the rear wheel of the tractor and the front wheel of the tractor, respectively;

xr _i ; yr _i

- coordinates corresponding to the points of application of the forces that act on the multiple bodies that make up the implement;

xπ; yπ

- coordinates of the instantaneous center of rotation of the suspension mechanism;

- horizontal coordinate of the central position of the tillage implement support wheel;

x _ga

- horizontal coordinate of the center of gravity of the tillage implement;

x _t ; x _dt ; x _gt

- coordinates of different characteristic points of the tractor.

To be able to carry out a stability analysis of the aggregate, it is necessary to determine the values of the vertical reactions that act on the wheels of the tractor. To do this, a quasi-static analysis is carried out, considering that the aggregate moves at a constant speed, which allows applying the equations of static equilibrium of the forces and moments in the x-y plane, that is:

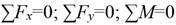
For the analysis, it is assumed that a study of the implement has been carried out, where the suction conditions have been guaranteed. Therefore, the resultant of the forces Rxy, Qa and Ga pass through the instantaneous center of rotation (point π) of the suspension system and the resultant of the forces Ga and Rxy, cause a moment in the direction of deepening of the tillage unit (clockwise in Figure 2). It is also based on the knowledge of the magnitudes, directions and application points of these forces. The geometrical characteristics of the tractor and its weight and position of the center of gravity are also known.

The unknown object of determination for the analysis are the reactions in the front wheels (Qd), in the rear wheels (Qt) and in the support wheels (Qa). It is also necessary to check the condition of penetration or suction of the implement.

As a starting point, it is necessary to determine the loads on the tillage units, which can be calculated knowing the specific resistance of that type of tillage units. In the case of the tillage units of staggered shanks, they are composed of wedges with “V”- shaped blades. Wedges with “V”- shaped blades have been studied by Albóniga (2015), with data on the specific resistance of this type of tillage unit in Ferrallitic-red type soils. Hence, if this indicator and the transversal area of soil that removes each body are known, it is feasible to determine the force of draft corresponding to each tillage unit, by means of the expression:

(1)

where:

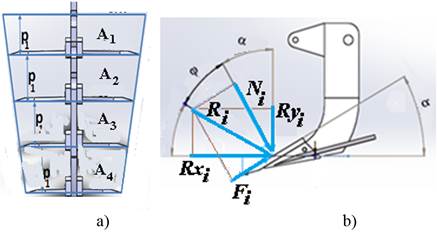
Rxi

- Horizontal component of the force of draft corresponding to the I^th tillage unit, N

- Specific resistance coefficient, N/m²

- Cross section of the soil section corresponding to the I^th tillage unit, m²

The areas Ai are determined on the basis of the geometry of the implements (Figure 3a).

FIGURE 3

a) Front view of the implement; (b) side view of one of the tillage units.

The vertical component and the resultant of the pulling force in each tillage unit are determined by the trigonometric expressions:

(2)

(3)

where:

- angle of the wedge with the horizontal

- sliding friction angle wedge-soil

The rolling friction forces on the support (F), front (Fd) and rear wheels of the tractor (Ft) are related to the normal reactions through the respective rolling resistance coefficients (µ _a , µ _d and µ _t ):

(4)

(5)

(6)

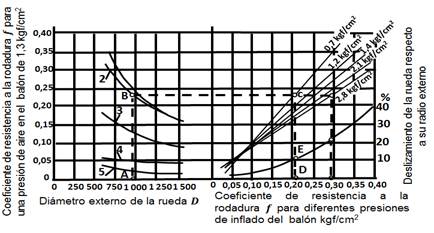
The coefficient of rolling resistance depends on the type and characteristics of the soil, the dimensions and characteristics of the wheels and their inflation pressure, among other factors. In Figure 4, a nomogram is provided that makes it possible to determine with sufficient approximation this coefficient for the case of rubber wheels (Sablikov, 1978).

FIGURE 4

Coefficient of resistance to rolling and sliding of pneumatic wheels: 1-loose sandy soil; 2-carved semiarcillous soil; 3-sowing soil; 4-harvested soil; 5-asphalt. Source: (Sablikov, 1978).

The vertical reaction in the support wheels is determined by taking moments in relation to the instantaneous center of rotation (π) of the suspension mechanism (Figure 2):

(7)

(8)

The condition of penetration or suction of the implement is determined taking moments with respect to the instantaneous center of rotation π of the forces Ga and Rxyi and checking its positive direction (in favor of the clockwise in Figure 2):

(9)

In order to determine the vertical reaction in the rear wheel of the tractor, the balance of moments with respect to the point of support of the front wheel (O`) and Nt is clarified:

(10)

Once Na and Nd are known, then the vertical reaction in the front tires (Nd) is determined, proposing the balance of forces in the vertical axis:

(11)

The horizontal component of the total draft force of the aggregate Pt is determined by raising the balance of forces in the direction of the x-axis:

(12)

The components Rxi, Fa, Fd and Ft are determined from expressions 1, 4, 5 and 6.

The draft force demanded by the implement, without considering the draft required by the tractor itself, is determined as:

(13)

where:

(14)

(15)

The angle that forms the draft force of the implement with the horizontal will be given by:

(16)

The set of developed equations were programmed using the software Mathcad 2000 Professional, obtaining the behavior of the different parameters depending on the position of the support wheels of the implement, as well as the position of the instantaneous center of rotation π and the angle of the wedges of the implement. The program was evaluated by entering the following data, which correspond to the aggregate under study:

Average specific resistance: Ke = 90 kN/m²;
Transverse area of soil corresponding to each tillage unit:

Angle of sliding friction soil-steel: φ = 30.96⁰;
Angle of the wedges with the horizontal: α = 20⁰; 30⁰; 40⁰;
Weight of the implement: Ga = 3.468 kN;
Weight of the tractor: Gt = 71.15 kN;
Coefficients of rolling resistance of the wheels :

µ _a = 0.24; µ _d = 0.17; µ _t = 0.14;

Wheel radius: ra = 0.158 , rt = 0.875 y rd = 0.656
Horizontal coordinate of the center position of the implement support wheel:

x = 0 … 2 m;

Coordinates of the rotation instant center of the suspension mechanism:

x _π = 0.60; 1.20; 1.80 m; y _π = 0.25; 0.50; 0.75 m;

Coordinates corresponding to the application points of the forces:

Coordinates of different characteristic points of the aggregate:

x _t = 1.338 m; x _dt = 2.887 m; x _gt = 1.094 m; x _ga = 0.525 m;

ANALYSIS OF THE RESULTS

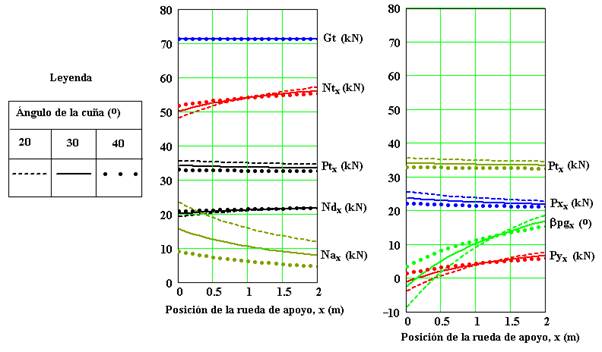
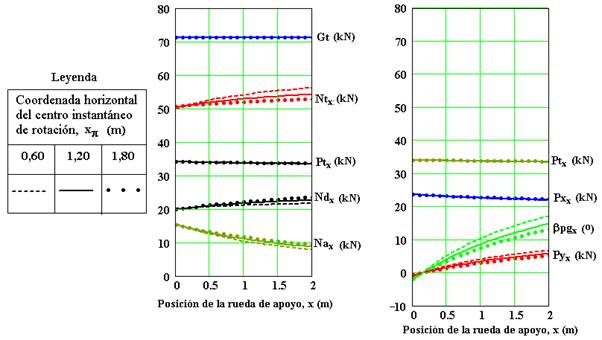
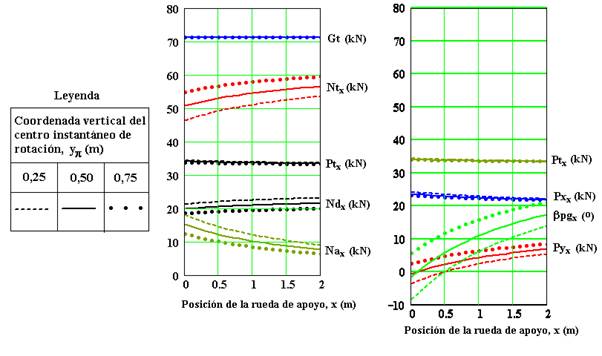
As a result of the evaluation of the system of equations developed, aided by its computer programming in Mathcad 2000 Professional, the behavior of the main output parameters was obtained according to the position of the support wheels of the implement, the angle of the wedge and of the position of the instantaneous center of rotation of the suspension system, which is shown in graphic form in Figures 5, 6 and 7.

FIGURE 5

Variation of the different parameters depending on the position of the support wheel and the angle of the wedge.

FIGURE 6

Variation of the different parameters as a function of the support wheel position and the horizontal coordinate (x_p) of the instantaneous rotation center of the suspension system.

FIGURE 7

Variation of the different parameters depending on the support wheel position and the vertical coordinate (y_p) of the instantaneous rotation center of the suspension system.

From the graphs, it can be seen that, as the center of the support wheels moves away with respect to the attachment points of the suspension system, the magnitude of the vertical ( Py ) component of the draft force and its angle ( βp ) with respect to the horizontal are increased in all cases. That affects the increase of the vertical reaction ( Nt ) on the rear wheels of the tractor, at the expense of a discharge of the support wheels of the implement, decreasing Na. In all cases there is also an increase, although slight, in the vertical load (Nd) on the front wheels of the tractor. It is of interest to note that, for positions of the support wheel very close to the suspension points to the tractor, the vertical component Py of the draft force can change sign and the angle βp can be negative, losing the suction condition or penetration of the implement. This situation is more pronounced for smaller values of the angle α of the wedge and for a small height and π of the instantaneous center of rotation of the suspension mechanism. It also highlights the fact that the horizontal position xπ of the instantaneous center of rotation (Fig. 6) has less influence on the variation of the different parameters evaluated, compared to the vertical position yπ, whose variation causes significant variations in the values of the vertical reactions of the soil on the wheels, both of the tractor, and of the implement. The equipment studied presents, as a proposal of the designers, a position of the support wheel x = 1.60 m, as well as an angle of the wedge α = 30⁰, which is considered acceptable according to the analyses carried out. From Figure 5, it is observed that in this case, adequate values of the vertical load Nt are obtained in the rear wheels, in the order of 56 kN, sufficient to guarantee power transmission during rolling under normal soil conditions. On the other hand, the load on the front wheels (of the order of 10 kN in each wheel), makes it possible to guarantee a stable direction of the tractor. Likewise, it is appreciated that in this case, the vertical load on the implement support wheel does not reach values of the lowest, in the evaluated range and that at the same time an adequate suction of the implement is guaranteed, being Py of the order of 6 kN and βp close to 150.

CONCLUSIONS

A mechanic-mathematical model of the system of forces acting on an aggregate formed by a tractor with rubber wheels with rear traction and a tillage implement having four staggered shanks with “V”- shaped blades is elaborated. Equations obtained were programmed based on Mathcad 2000 Professional software, which allows replacing the cumbersome traditional method of constructing force polygons and rays. As result of the model application, the influence of the position of the support wheels of the implement, the angle of the wedges of the tillage units and the position of the instantaneous center of rotation of the tractor suspension system on output parameters is obtained. Such parameters are the vertical reactions of the soil on the tractor wheels and the support wheel of the implement, as well as the magnitude and direction of the draft force, allowing evaluating the conditions of stability of the aggregate and suction of the implement. The variation of the vertical position y_π of the instantaneous center of rotation of the suspension mechanism caused the most significant variations in the values of the vertical reactions of the ground on the wheels, both of the tractor, and of the implement. Likewise, it was determined that for positions of the support wheel very close to the points of suspension to the tractor, a change of sign occurs in the vertical component Py of the draft force, losing the condition of suction or penetration of the implement. This situation is more pronounced for smaller values of the angle α of the wedge and for a small height and y _π of the instantaneous center of rotation of the suspension mechanism.

INTRODUCCIÓN

La caña de azúcar (Saccharum officinarum), se cultiva en las regiones tropicales y subtropicales, creciendo significativamente las exportaciones entre los años 2000 y 2011, fundamentalmente en Brasil, que pasó de exportar 6 500 000 t en 2000 a 25 500 000 t en 2011, siendo el mayor exportador mundial de azúcar, seguido por México, Guatemala y Colombia. En el año 2000 Cuba era el segundo mayor exportador de azúcar en la región, registrando 3 500 000 t, cayendo en 2011 a 648 000 t según FAO (2014), manifestándose en los últimos años una disminución significativa de la producción de azúcar, entre otros factores, debido a los bajos rendimientos agrícolas, por lo que se ha considerado necesario realizar cambios en las prácticas agrícolas actuales (Herrera et al., 2003; Leyva et al., 2007; Betancourt et al., 2008). Entre las que se ejecuta el cambio del marco de siembra tradicional al de surcos de doble hilera (Romer et al., 2009; Gómez et al., 2011; Ullah et al., 2016; Guru et al., 2017). Paralelamente se desarrollan equipos que se adapten a estos nuevos sistemas de plantación, incluyendo los destinados a la preparación de los suelos para la siembra.

Cruz et al. (2018) plantean que en la actualidad las tecnologías que se emplean para la preparación del suelo son: la tecnología convencional y la conservacionista. Esta última se basa fundamentalmente en la preparación localizada del suelo efectuada exclusivamente en la zona de crecimiento radicular de los plantones, constituyendo una alternativa viable, tanto desde el punto de vista de la conservación del suelo, como de economía de recursos energéticos que se dedican a la preparación de grandes extensiones de tierra.

Como parte del proyecto nacional Incremento de la efectividad de los procesos mecanizados en la producción de caña de azúcar en la Unidad Empresarial de Base “Héctor Molina Riaño”, perteneciente al programa: Desarrollo de la Agroindustria Azucarera, que ejecuta el Centro de Mecanización Agropecuaria (CEMA) de la Universidaad Agraria de La Habana, se realiza la tarea: Desarrollo y evaluación de apero de preparación localizada del suelo, requiriéndose durante el proceso de diseño de este equipo, efectuar determinados análisis relacionados con la succión y estabilidad, para lo cual se ha desarrollado un método analítico y un software que facilita estos análisis, evitando el empleo de engorrosos métodos gráficos tradicionales, basados en la elaboración de polígonos de fuerza y de rayos, que dificultan la evaluación de múltiples variantes.

En el presente trabajo se expone el método y el software desarrollados, a través de su aplicación en un estudio de caso del agregado formado por un tractor de ruedas de goma YTO-1604 y un escarificador escalonado de cuatro cuerpos que se desarrolla para la preparación localizada del suelo destinado a la siembra de caña de azúcar en surcos de doble hilera.

MÉTODOS

Fundamentación teórico-metodológica

Para desarrollar el método analítico de cálculo y el software correspondiente, se partió de la modelación mecánico-matemática de la interacción del agregado con el suelo, aplicando las leyes de la mecánica al sistema tractor-apero-suelo. En la Figura 1 se muestra una vista en isométrico del escarificador objeto de estudio, donde puede apreciarse el escalonamiento de cuatro cuerpos escarificadores con saetas que laboran a diferente profundidad, siguiendo el principio de la brocha mecánica (Albóniga, 2011, 2015; Domínguez et al., 2014).

FIGURA 1

Representación en isométrico del escarificador escalonado objeto de estudio.

Las condiciones de estabilidad se evalúan determinando las cargas que actúan sobre las ruedas del tractor, tanto las traseras, como las delanteras. En este caso se efectúa un análisis correspondiente a un tractor con tracción en las ruedas traseras. Es preciso aclarar que el análisis efectuado corresponde al plano longitudinal-vertical (x-y).

En la Figura 2 se muestra el sistema de fuerzas que actúan sobre el agregado formado por un tractor YTO-1604 de ruedas de goma con tracción en las ruedas traseras y el escarificador escalonado.

FIGURA 2

Sistema de fuerzas que actúan en el agregado tractor-escarificador escalonado.

Para el logro de un buen “agarre” o adhesión de las ruedas traseras, que posibilite la transmisión de la potencia demandada en su interacción con el suelo, se requiere una determinada fuerza de reacción normal ( Nt ) en la rueda trasera o motriz (Figura 2). Si esta fuerza no alcanza un valor suficiente, entonces puede producirse el patinaje de las ruedas y no transmitirse la componente horizontal ( Pt ) de la fuerza de tiro requerida para el trabajo del implemento agregado al tractor. En este caso el trabajo del agregado se considera inestable.

Claro está que existen otros parámetros que influyen en la capacidad de la rueda motriz para transmitir la potencia requerida a través del suelo, entre los que se encuentran la adherencia del propio suelo y su estado de compactación y humedad, así como el propio diseño de los neumáticos.

Por otro lado, con relación a las ruedas delanteras, para garantizar una buena dirección del tractor, se requiere una carga ( Nd ) adecuada sobre dichas ruedas, que no debe ser excesiva, pero tampoco insuficiente, pues en este caso pudiera dificultarse el control de la dirección del tractor y en el caso extremo, producirse el levantamiento de las ruedas delanteras.

Un trabajo estable del agregado se complementa con una adecuada “succión” del apero que garantice la penetración natural de los órganos escarificadores en el suelo con el sistema de suspensión trabajando en posición flotante.

El modelo que se presenta es implementado de forma tal que facilite la evaluación de la influencia de diferentes parámetros sobre las características de succión y estabilidad del sistema. Entre estos parámetros que se toman como variables de entrada, se encuentran la posición de la rueda de apoyo, el ángulo de las cuñas que conforman el escarificador y la posición del centro instantáneo de rotación del sistema de suspensión del tractor (punto π en la Figura 2), que a la vez constituyen elementos de diseño o regulación del equipo.

Las fuerzas y coordenadas que aparecen en la Figura 2 son las siguientes:

- Fuerza de tiro requerida para el movimiento del agregado;

β _p

- es el ángulo que forma con la horizontal la resultante P de la resistencia a la tracción del escarificador;

- Componente horizontal de la fuerza de tiro;

Rx _i y Ry _i

- son las componentes de la fuerza de resistencia que actúa en cada órgano;

Rxy

- es la resultante de las fuerzas de resistencia del suelo a la acción de los órganos de trabajo;

Qa, Qt y Qd

- son las reacciones del suelo sobre la rueda de apoyo del implemento, la rueda trasera del tractor y la rueda delantera del tractor, respectivamente;

Fa, Ft y Fd

- son las componentes horizontales (fricción por rodadura) de las reacciones Qi ;

Na, Nt y Nd

- son las componentes normales de las reacciones Qi ;

φa, φt y φd

- son los ángulos de resistencia a la rodadura de la rueda de apoyo del implemento, la rueda trasera del tractor y la rueda delantera del tractor, respectivamente;

Ga y Gt

- son los pesos del apero y del tractor, respectivamente;

ra, rt y rd

- son los radios de la rueda de apoyo del implemento, la rueda trasera del tractor y la rueda delantera del tractor, respectivamente;

xr _i ; yr _i

- son las coordenadas correspondientes a los puntos de aplicación de las fuerzas que actúan sobre los múltiples cuerpos que componen el escarificador;

xπ; yπ

- son las coordenadas del centro instantáneo de rotación del mecanismo de suspensión;

- es la coordenada horizontal de la posición del centro de la rueda de apoyo del apero;

x _ga

- es la coordenada horizontal del centro de gravedad del apero;

x _t ; x _dt ; x _gt

- son las coordenadas de diferentes puntos característicos del tractor.

Para poder efectuar un análisis de estabilidad del agregado, se requiere determinar los valores de las reacciones verticales que actúan sobre las ruedas del tractor. Para ello se efectúa un análisis quasi-estático, considerando que el agregado se desplaza a velocidad constante, lo que permite aplicar las ecuaciones de equilibrio estático de las fuerzas y momentos en el plano x-y, o sea:

∑F _x =0; ∑F _y =0; ∑M=0

Para el análisis se parte de que se ha efectuado previamente un estudio del implemento, donde se ha garantizado las condiciones de succión, de manera que la resultante de las fuerzas Rxy , Qa y Ga pase por el centro instantáneo de rotación (punto µ) del sistema de suspensión y que la resultante de las fuerzas Ga y Rxy , provoquen un momento en el sentido de profundización de los órganos de trabajo (sentido de las agujas del reloj en la Figura 2). Asimismo se parte del conocimiento de las magnitudes, direcciones y puntos de aplicación de dichas fuerzas. También son conocidas las características geométricas del tractor y su peso y posición del centro de gravedad.

Las incógnitas objeto de determinación para el análisis son las reacciones en las ruedas delanteras ( Qd ), en las ruedas traseras ( Qt ) y en las ruedas de apoyo ( Qa ). Asimismo es necesario comprobar la condición de penetración o succión del implemento.

Como punto de partida es necesario determinar las cargas sobre los órganos de trabajo, las cuales pueden calcularse conociendo la resistencia específica de ese tipo de órganos. En el caso de los órganos escarificadores escalonados, los órganos de trabajo están compuestos de cuñas con saetas. Los órganos de cuña con saetas han sido estudiados por Albóniga (2015), contándose con datos sobre la resistencia específica de este tipo de órgano en suelos del tipo ferralíticos-rojo, de manera que conociendo este indicador y el área transversal de suelo que remueve cada cuerpo, es factible determinar la fuerza de tiro correspondiente a cada órgano de trabajo, mediante la expresión:

(1)

donde:

Rxi

- Componente horizontal de la fuerza de tiro correspondiente al órgano iésimo, N;

- Coeficiente de resistencia específica; N/m²;

- Área transversal de la sección del suelo correspondiente al órgano iésimo, m².

Las áreas Ai se determinan sobre la base de la geometría de los implementos (Figura 3a).

FIGURA 3

a) Vista frontal del implemento; (b) vista lateral de uno de los órganos.

La componente vertical y la resultante de la fuerza de tiro en cada órgano se determinan mediante las expresiones trigonométricas:

(2)

(3)

donde:

- ángulo de la cuña con la horizontal;

- ángulo de fricción por deslizamiento cuña-suelo.

Las fuerzas de fricción por rodadura en las ruedas de apoyo ( Fa ), delanteras ( Fd ) y traseras del tractor ( Ft ) están relacionadas con las reacciones normales a través de los coeficientes de resistencia a la rodadura respectivos (µ _a , µ _d y µ _t ):

(4)

(5)

(6)

El coeficiente de resistencia a la rodadura depende del tipo y características del suelo, de las dimensiones y características de las ruedas y su presión de inflado, entre otros factores. En la Figura 4, se brinda un nomograma que posibilita determinar con suficiente aproximación este coeficiente para el caso de ruedas de goma (Sablikov, 1978).

FIGURA 4

Coeficiente de resistencia a la rodadura y deslizamiento de las ruedas neumáticas: 1-suelo arenoso suelto; 2-suelo semiarcilloso labrado; 3-suelo de sembradío; 4-suelo cosechado; 5-asfalto. Fuente: Sablikov, (1978).

La reacción vertical en las ruedas de apoyo se determinan tomando momentos con relación al centro instantáneo de rotación (π) del mecanismo de suspensión (Figura 2):

(7)

(8)

La condición de penetración o succión del implemento se determina tomando momentos respecto al centro instantáneo de rotación π de las fuerzas Ga y Rxy _i y comprobando su sentido positivo (a favor de las agujas del reloj en la Figura 2):

(9)

Para determinar la reacción vertical en la rueda trasera del tractor se plantea el equilibrio de momentos con respecto al punto de apoyo de la rueda delantera (O`) y se despeja Nt :

(10)

Una vez conocidos Na y Nd, entonces se determina la reacción vertical en los neumáticos delanteros (Nd), planteando el equilibrio de fuerzas en el eje vertical:

(11)

La componente horizontal de la fuerza total de tiro del agregado Pt se determina planteando el equilibrio de fuerzas en la dirección del eje x:

(12)

Los componentes Rx_i , Fa, Fd y Ft se determinan de las expresiones 1, 4, 5 y 6.

La fuerza de tiro demandada por el apero, sin considerar el tiro requerido por el propio tractor, se determina como:

(13)

donde:

(14)

(15)

El ángulo que forma la fuerza de tiro del apero con la horizontal estará dada por:

(16)

El conjunto de ecuaciones desarrolladas, fueron programadas empleando el software Mathcad 2000 Professional, obteniéndose el comportamiento de los diferentes parámetros en función de la posición de las ruedas de apoyo del apero, así como de la posición del centro instantáneo de rotación π y del ángulo de las cuñas del escarificador. El programa fue evaluado introduciendo los siguientes datos, que corresponden al agregado objeto de estudio:

Resistencia específica media: Ke = 90 kN/m²;
Área transversal del suelo correspondiente a cada órgano:

Ángulo de fricción por deslizamiento suelo-acero: φ = 30.96⁰;
Ángulo de las cuñas con la horizontal: α = 20⁰; 30⁰; 40⁰;
Peso del apero: Ga = 3.468 kN;
Peso del tractor: Gt = 71.15 kN;
Coeficientes de resistencia a la rodadura de las ruedas :

µ _a = 0.24; µ _d = 0.17; µ _t = 0.14

Radios de las ruedas: ra = 0.158 , rt = 0.875 y rd = 0.656
Coordenada horizontal de la posición del centro de la rueda de apoyo del apero:

x = 0 … 2 m;

Coordenadas del centro instantáneo de rotación del mecanismo de suspensión:

x _π =0.60; 1.20;1.80 m; y _π = 0.25; 0.50; 0.75 m;

Coordenadas correspondientes a los puntos de aplicación de las fuerzas:

Coordenadas de diferentes puntos característicos del agregado:

x _t = 1.338 m; x _dt = 2.887 m; x _gt = 1.094 m; x _ga = 0.525 m;

ANÁLISIS DE LOS RESULTADOS

Como resultado de la evaluación del sistema de ecuaciones desarrollado, auxiliados por su programación computarizada en Mathcad 2000 Professional, se obtuvo el comportamiento de los principales parámetros de salida en función de la posición de las ruedas de apoyo del apero, del ángulo de la cuña y de la posición del centro instantáneo de rotación del sistema de suspensión, lo cual se muestra en forma gráfica en las Figuras 5, 6 y 7.

FIGURA 5

Variación de los diferentes parámetros en función de la posición de la rueda de apoyo y del ángulo de la cuña.

FIGURA 6

Variación de los diferentes parámetros en función de la posición de la rueda de apoyo y de la coordenada horizontal (x_p) del centro instantáneo de rotación del sistema de suspensión.

FIGURA 7

Variación de los diferentes parámetros en función de la posición de la rueda de apoyo y de la coordenada vertical (y_p) del centro instantáneo de rotación del sistema de suspensión.

De los gráficos se aprecia que a medida que se aleja el centro de las ruedas de apoyo con respecto a los puntos de enganche del sistema de suspensión, se incrementa en todos los casos, la magnitud de la componente vertical ( Py ) de la fuerza de tiro y su ángulo ( βp ) con respecto a la horizontal, lo que repercute en el incremento de la reacción vertical ( Nt ) en las ruedas traseras del tractor, a expensas de una descarga de las ruedas de apoyo del apero, disminuyendo Na . También se aprecia en todos los casos un incremento, aunque ligero, de la carga vertical ( Nd ) sobre las ruedas delanteras del tractor.

Es de interés notar que, para posiciones de la rueda de apoyo muy cercanas a los puntos de suspensión al tractor, puede cambiar de signo la componente vertical Py de la fuerza de tiro y ser negativo el ángulo βp , perdiéndose la condición de succión o penetración del apero. Esta situación es más acentuada para valores menores del ángulo α de la cuña y para una pequeña altura y _π del centro instantáneo de rotación del mecanismo de suspensión.

Asimismo se destaca el hecho de que la posición horizontal x _π del centro instantáneo de rotación (Fig. 6) presenta menos influencia en la variación de los diferentes parámetros evaluados, si se compara con relación a la posición vertical y _π , cuya variación provoca variaciones significativas en los valores de las reacciones verticales del suelo sobre las ruedas, tanto del tractor, como del apero.

El equipo objeto de estudio presenta, como propuesta de los diseñadores, una posición de la rueda de apoyo x = 1.60 m, así como un ángulo de la cuña α = 30⁰, lo cual se considera aceptable de acuerdo a los análisis realizados. De la Figura 5 se observa que en este caso se obtienen adecuados valores de la carga vertical Nt en las ruedas traseras, del orden de los 56 kN, suficiente para garantizar la transmisión de potencia durante la rodadura en condiciones normales del suelo. Por otro lado, la carga sobre las ruedas delanteras (del orden de los 10 kN en cada rueda), posibilita garantizar una dirección estable del tractor. Asimismo se aprecia que en este caso, la carga vertical sobre la rueda de apoyo del apero no alcanza valores de los más bajos, en el rango evaluado y que a su vez se garantiza una succión adecuada del implemento, siendo Py del orden de los 6 kN y βp cercano a los 15⁰.

CONCLUSIONES

Se elabora un modelo mecánico-matemático del sistema de fuerzas que actúan sobre un agregado formado por un tractor de ruedas de goma con tracción trasera y un escarificador escalonado de cuatro cuerpos, programándose las ecuaciones obtenidas sobre la base del software Mathcad 2000 Professional, lo que permite sustituir el engorroso método tradicional de construcción de polígonos de fuerza y de rayos.

Como resultado de la aplicación del modelo, se obtiene la influencia de la posición de las ruedas de apoyo del apero, del ángulo de las cuñas de los escarificadores y de la posición del centro instantáneo de rotación del sistema de suspensión del tractor sobre parámetros de salida tales como: las reacciones verticales del suelo sobre las ruedas del tractor y la rueda de apoyo del apero, así como la magnitud y dirección de la fuerza de tiro, permitiendo evaluar las condiciones de estabilidad del agregado y de succión del apero.

La variación de la posición vertical y _π del centro instantáneo de rotación del mecanismo de suspensión, provocó las variaciones más significativas en los valores de las reacciones verticales del suelo sobre las ruedas, tanto del tractor, como del apero.

Asimismo se determinó que para posiciones de la rueda de apoyo muy cercanas a los puntos de suspensión al tractor, se produce un cambio de signo en la componente vertical Py de la fuerza de tiro, perdiéndose la condición de succión o penetración del apero. Esta situación es más acentuada para valores menores del ángulo α de la cuña y para una pequeña altura y _π del centro instantáneo de rotación del mecanismo de suspensión.

Analysis of Suction and Stability of Implement in Localized Soil Preparation for Sugarcane Planting

REFERENCES

Análisis de succión y estabilidad en apero para la preparación localizada del suelo para caña de azúcar